👉 View PDF
Chapter 8: FORCE AND LAWS OF MOTION
The speed of an object changes over time due to the application of a force. It causes the object to accelerate, decelerate, or alter its direction of motion.
Earlier, scientists believed rest was the natural state of objects. This view changed when Galileo Galilei and Isaac Newton suggested that an object in motion stays in motion unless acted upon by a force.
Force is a push, pull, or hit that causes a change in an object's state of motion. It is not visible, but its effects are observable. E.g., trolley moves when pushed, pulling a drawer, hockey stick hits the ball forward etc.
A force can
· Change velocity of an object (move faster or slower).
· Change the direction of motion of an object.
· Change the shape and size of objects. (e.g., squashing or stretching).
(a) A spring expands on application of force;
(b) A rubber ball becomes oblong as we apply force on it.
BALANCED AND UNBALANCED FORCES
- Consider a wooden block is positioned on a table with two strings, X and Y, tied to opposite sides. Pulling string X moves the block right, and pulling Y moves it left.
- When equal forces are applied to both strings, the block remains stationary. These are called balanced forces. They do not alter the object's state of rest or motion.
- If unequal forces are applied from both sides, the block moves in the direction of the greater force. They are called unbalanced forces which cause an object to move.
As the pushing force increases, friction force continues to balance it, and the box still does not move [Fig. b).
When the pushing force exceeds the friction force, an unbalanced force is created, and the box moves. (Fig. c).
A bicycle slows down when pedaling stops due to friction forces acting against its motion. To keep it moving, pedaling must restart, but continuous unbalanced force is not necessary to maintain motion.
When an object moves with uniform velocity, the forces acting on it (pedalling force and frictional force) are balanced, with no net external force.
Unbalanced forces change an object's speed or direction, causing acceleration. Once the force is removed, the object continues moving with the velocity it had acquired up to that point.
FIRST LAW OF MOTION
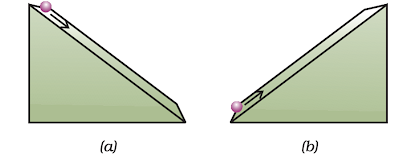
Galileo's experiment with a marble on an inclined plane demonstrated that objects move at a constant speed when no force acts on them.
When a marble rolls down an incline, its velocity increases due to the unbalanced force of gravity. As the marble climbs up another incline, its velocity decreases.
In an ideal frictionless setup, the marble would roll up to the same height it was released from, if the inclines are equal on both sides.
Galileo's conclusion: If there is no unbalanced force, like on a flat surface, the marble would continue to move forever at a uniform speed, as no force is required to maintain motion.
In practice, objects slow down due to friction. Reducing friction (e.g., using a smooth surface or lubricants) can minimize this effect.
Newton further studied Galileo’s work and presented three fundamental laws that explain the motion of objects. These are known as Newton’s laws of motion.
The first law of motion (Law of inertia):
An object remains in a state of rest or of uniform motion in a straight line unless compelled to change that state by an applied force.
i.e., all objects resist a change in their state of motion.
The tendency of undisturbed objects to stay at rest or to keep moving with the same velocity is called inertia. E.g.,
· When a car suddenly brakes, our body continues moving forward due to inertia, which can lead to injury. Safety belts prevent this by exerting a force on our body that slows forward motion.
· When a bus starts moving suddenly, we tend to fall backward due to inertia. The sudden motion of the bus moves feet, which are in contact with the floor. But the rest of our body opposes this motion because of its inertia.
When a motorcar makes a sharp turn at high speed, the body tends to maintain its motion in a straight line due to inertia. The car's change in direction applies an unbalanced force, but the body's inertia resists this change, causing a sensation of being thrown to one side.
The body remains at rest unless acted upon by an unbalanced force. It is shown through some activities:
• Activity 1: Hit the bottom carom coin in a pile. The bottom coin moves, and the others fall vertically due to inertia.
• Activity 2: Flick a card holding a coin over a glass. The card moves and the coin falls into the glass due to inertia.
• Activity 3: Spin a tray with a water-filled tumbler. The water spills due to inertia. A groove in a saucer helps prevent the cup from toppling during jerks.
INERTIA AND MASS
Inertia is not the same for all bodies. Heavier or more massive objects have larger inertia. So they resist changes of rest or motion more strongly. E.g.,
· It is easier to push an empty box than a box full of books.
· If we kick a football, it flies away. But if we kick a stone of the same size, it hardly moves.
· In activity 2, using a one-rupee coin instead of a five-
rupee coin requires less force.
· A force sufficient to give a small cart a large velocity will have a negligible effect on a train's motion due to the train's larger inertia.
Inertia is the natural tendency of an object to resist a change in its state of motion or of rest. Quantitatively, the inertia of an object is measured by its mass.
SECOND LAW OF MOTION
First law of motion states that an unbalanced external force changes an object's velocity, causing acceleration.
The acceleration of an object depends on the force.
The impact of objects depends on their mass and velocity. E.g., A table tennis ball hitting a player is not painful, while a fast-moving cricket ball can hurt. A truck at rest is not a threat, but even a slow-moving truck can be dangerous. A small mass, like a bullet can kill a person when fired from a gun.
To accelerate an object, a greater force is needed for a higher velocity. This led Newton to introduce the concept of momentum. Momentum (p) is defined as the product of an object's mass, m and velocity, v.
p = mv
Momentum has both direction and magnitude. Its direction is the same as that of velocity.
SI unit of momentum: kg m s-1.
Since an unbalanced force changes an object's velocity, it also results in a change in momentum.
When pushing a car with a dead battery, a sudden push (unbalanced force) does not result in enough speed to start the engine. However, a continuous push over time gradually accelerates the car to the required speed of 1 m/s. This shows that the change in the car’s momentum depends not only on the force but also on the duration (time) of the force. Also, the force necessary to change the momentum of an object depends on the time rate at which the momentum is changed.
Second law of motion states that the rate of change of momentum of an object is proportional to the applied unbalanced force in the direction of force.
MATHEMATICAL FORMULATION OF SECOND LAW OF MOTION
Suppose an object of mass, m is moving along a straight line with an initial velocity, u. It is uniformly accelerated to velocity, v in time, t by the application of a constant force, F throughout the time, t.
The initial and final momentum of the object will be, p1 = mu and p2 = mv respectively.
The change in momentum ∝ p2 – p1
∝ mv – mu
∝ m × (v – u)
One unit of force is defined as the amount that produces an acceleration of 1 m s-2 in an object of 1 kg mass.
i.e., 1 unit of force = k × (1 kg) × (1 m s-2).
Thus, the value of k becomes 1.
F = ma
- The unit of force is kg m s-2 or newton (N).
- Second law of motion gives a method to measure the force as a product of its mass and acceleration.
The second law of motion in daily life
· When catching a fast-moving cricket ball, a fielder pulls his hands backward, increasing the time over which the ball's velocity decreases to zero. This reduces the ball's acceleration and impact. Stopping the ball suddenly would require a large force, causing injury.
· In high jump events, athletes land on cushioned or sand beds to extend the time of their fall, decreasing the rate of change of momentum and the force of impact.
· A karate player breaks a slab of ice with a single blow by concentrating a large amount of force on a small area, causing the ice to break.
i.e., when F = 0, v = u for any time, t. This means that the object will continue moving with uniform velocity, u. If u is zero, v will also be zero. i.e., the object remains at rest.
Example: A constant force acts on an object of mass 5 kg for a duration of 2 s. It increases the object’s velocity from 3 m s–1 to 7 m s-1. Find the magnitude of the applied force. Now, if the force was applied for a duration of 5 s, what would be the final velocity of the object?
Solution:
u = 3 m s–1 v = 7 m s-1 t = 2 s m = 5 kg
F = 5 kg (7 m s-1 – 3 m s-1)/2 s = 10 N
If this force is applied for a duration of 5 s (t = 5 s), then the final velocity is
v= 3 + 10 x 5/5 = 13 m s-1
Example: Which would require a greater force - accelerating a 2 kg mass at 5 m s–2 or a 4 kg mass at 2 m s-2?
Solution:
F = ma
m1 = 2 kg; a1 = 5 m s-2
m2 = 4 kg; a2 = 2 m s-2.
F1 = m1a1 = 2 kg × 5 m s-2 = 10 N
F2 = m2a2 = 4 kg × 2 m s-2 = 8 N
⇒ F1 > F2.
So, accelerating a 2 kg mass at 5 m s-2 needs a greater force.
Example: A motorcar is moving with a velocity of 108 km/h and it takes 4 s to stop after the brakes are applied.
Calculate the force exerted by the brakes on the motorcar if its mass along with the passengers is 1000 kg.
Solution:
Initial velocity, u = 108 km/h
= 108 × 1000 m/(60 × 60 s)= 30 m s-1
Final velocity, v = 0 m s-1.
Mass of the motorcar along with passengers = 1000 kg
Time taken to stop the motorcar, t = 4 s.
F= m(v – u)/t
F = 1000 kg × (0 – 30) m s-1/4 s
= – 7500 kg m s-2 or – 7500 N
The negative sign indicates that the braking force is opposite to the direction of motion of the motorcar.
Example: A force of 5 N gives a mass m1, an acceleration of 10 m s–2 and a mass m2, an acceleration of 20 m s-2.
What acceleration would it give if both the masses were tied together?
Solution:
m1 = F/a1 and m2 = F/a2.
a1 = 10 m s-2 a2 = 20 m s-2 F = 5 N
m1 = 5 N/10 m s-2 = 0.50 kg
m2 = 5 N/20 m s-2 = 0.25 kg
If the two masses were tied together, the total mass, m = 0.50 kg + 0.25 kg = 0.75 kg
The acceleration, a produced in the combined mass is
a = F/m = 5 N/0.75 kg = 6.67 m s-2.
Example: The velocity-time graph of a ball of mass 20 g moving along a straight line on a long table is given below:
How much force does the table exert on the ball to bring it to rest?
Solution:
The initial velocity of the ball is 20 cm s-1.
Due to the frictional force exerted by the table, the velocity of the ball decreases down to zero in 10 s.
Thus, u = 20 cm s–1; v = 0 cm s-1 and t = 10 s.
Since the velocity-time graph is a straight line, ball moves with a constant acceleration. The acceleration a is
= (0 cm s-1 – 20 cm s-1)/10 s
= –2 cm s-2 = –0.02 m s-2.
The force exerted on the ball is, F = ma
= (20/1000) kg × (– 0.02 m s-2) = – 0.0004 N.
The negative sign implies that the frictional force exerted by the table is opposite to the direction of ball’s motion.
THIRD LAW OF MOTION
The first two laws of motion explain how an applied force changes motion and provide a way to determine the force.
Third law of motion states that when one object exerts a force on another, the second object instantaneously exerts an equal and opposite force on the first.
These forces act on different objects, never on the same one. E.g., in football, when two players collide while aiming for the ball, both feel hurt because they exert forces on each other. This pair of opposing forces is known as action and reaction forces.
Consider two spring balances connected together. The fixed end of balance B is attached to a rigid support. When a force is applied through the free end of balance A, both spring balances show the same reading. This indicates that the force exerted by A on B is equal and opposite to the force exerted by B on A. Any of these forces can be called action, and the other reaction.
Thus, the third law of motion can be modified as for every action, there is an equal and opposite reaction. These forces act on different objects simultaneously.
When standing still and intending to start walking, acceleration requires a force according to the second law of motion. This force is not due to the muscular effort exerted on the road in the direction of movement. Instead, the road is pushed backward, and an equal and opposite force exerted by the road on the feet causes forward motion.
Although action and reaction forces are always equal in magnitude, they may not cause accelerations of equal magnitudes. This is because each force acts on a different object with a different mass.
When a gun is fired, it exerts a forward force on the bullet. The bullet exerts an equal and opposite force on the gun. This results in the recoil of the gun. Due to the gun's larger mass, its acceleration is much less than that of the bullet.
When a sailor jumps forward out of a rowing boat, the force exerted by the sailor moves the boat backward.
Activity
• Stand two children on two separate carts.
• Give them a bag full of sand or similar heavy object.
• Ask them to play catch with the bag. They experience an instantaneous force due to throwing the sand bag.
• Paint a white line on the cartwheels to observe the movement of the carts as the children throw the bag towards each other.
· Place two children on one cart and one on another. This setup demonstrates the second law of motion, showing different accelerations for the same force.
👉 View PDF